Whilst researching some new tutorial content for this site, I stumbled upon a topic which seems to have a lot of people confused, and I have to be honest that when I began delving deeper into it, initially I just became more and more confused myself.
The seemingly simple question is: Is there a difference between angle of view (AOV) and field of view (FOV)?
I realized that in the past I had used the two terms somewhat interchangeably, but I began to wonder if that was incorrect even though I found many other people doing the same thing, such as Bob Atkins, who refers to FOV when defining the equation for it, but labels the resulting graph with AOV.
The Wikipedia entry for field of view starts with the first line “For the same phenomenon in photography, see Angle of view” which certainly seems to indicate that the two are interchangeable. Unfortunately it quickly contradicts itself in the very first line of the angle of view entry by citing a source that clearly states that people should not treat FOV and AOV the same. That source, linked in the previous sentence, states that AOV is an angle, whilst FOV should be a measurement of linear distance.
That actually made a lot of sense to me, but other sources I trust explicitly on such matters, such as the excellent Photography Life website, contradict that statement by saying that whilst AOV and FOV are different things, they are both measured as angles. In their article it states that AOV is a property of the lens and does not change no matter what size of sensor is in the camera, whilst FOV is a function of the lens AND the sensor size. In other words, a full frame lens can have a particular AOV, but when used on a crop sensor camera the actual field of view (FOV) is going to be smaller. Once again, taken in a vacuum, this sounds like a perfectly excellent way to define both terms, but it does contradict other sources and I struggled to find anywhere else that suggested that particular pair of definitions.
I then started to look around to see how camera manufacturers were using the terminology and found that Canon, Nikon and Sony all cite angle of view in their lens specifications on their websites and appear to prefer this terminology over field of view. However, they also include the angle of view for both full frame lenses, and APS-C lenses (see example below), which is contrary to the usually excellently researched content on Photography Life which says that AOV for a lens is constant, and only FOV changes based on sensor size.
From the SONY website section on lens basics
“Angle of view” describes how much of the scene in front of the camera will be captured by the camera’s sensor. In slightly more technical terms, it is the angular extent of the scene captured on the sensor, measured diagonally. It is important to remember that angle of view is entirely determined by both the focal length of the lens and the format of the camera’s sensor, so the angle of view you get from any given lens will be different on 35mm full-frame and APS-C format cameras. Different lenses of equal focal length will always have the same angle of view when used with the same-size sensor
From the Nikon website on lens basics
The angle of view is the visible extent of the scene captured by the image sensor, stated as an angle. Wide angle of views capture greater areas, small angles smaller areas. Changing the focal length changes the angle of view. The shorter the focal length (e.g. 18 mm), the wider the angle of view and the greater the area captured. The longer the focal length (e.g. 55 mm), the smaller the angle and the larger the subject appears to be.
Nikon agrees with Sony here, although they do then go completely rogue and start talking about “picture angle” in a section labelled “angle of view” which is a term never mentioned anywhere else by anyone else, and, in my opinion is probably just the result of a poor translation at some point.
So What the Heck is AFOV?
At this point I was thoroughly puzzled by all of this and all signs were pointing to the fact that the two terms are just so similar to each other that the internet has completely befuddled itself about them. Then I started to notice a third term popping up in some content, such as this article by Edmund Optics which was referring to AFOV, as in angular field of view.
Ok, so now we have FOV, AOV and AFOV!
That particular article used the term AFOV in place of what I was coming to define as AOV. I can accept that, because when you think about the term field of view it doesn’t seem as though it should be an angle, so the addition of the word angular makes sense there if you are going to use an angle as the unit of measurement. In some cases I also found people referring to the term “linear field of view” which I think we can all agree is just a way of underlining that this is a distance rather than an angle.
Here’s How I am Going to Define FOV and AOV!
I have a feeling that there’s just that much confused information out there on this topic that people will discuss (and argue about) it forever, but I wasn’t willing to sit back until I had come up with a set of definitions that I was happy to adopt for use on this site and in my teachings going forwards. Feel free to disagree with me in the comments, I’m absolutely open to other suggestions if they can be backed up with some source of information. Having read countless arguments and blog posts on the topic though (seriously, I spent 8 hours reading about this today), I’m happy with how I have concluded to tackle these terms in the future. My definitions, as presented below, are the ones that make the most sense to me when I piece everything together.
So here is how I am going to define and use these terms on this site and in my future work unless someone can provide me with some well sourced information that contradicts this:
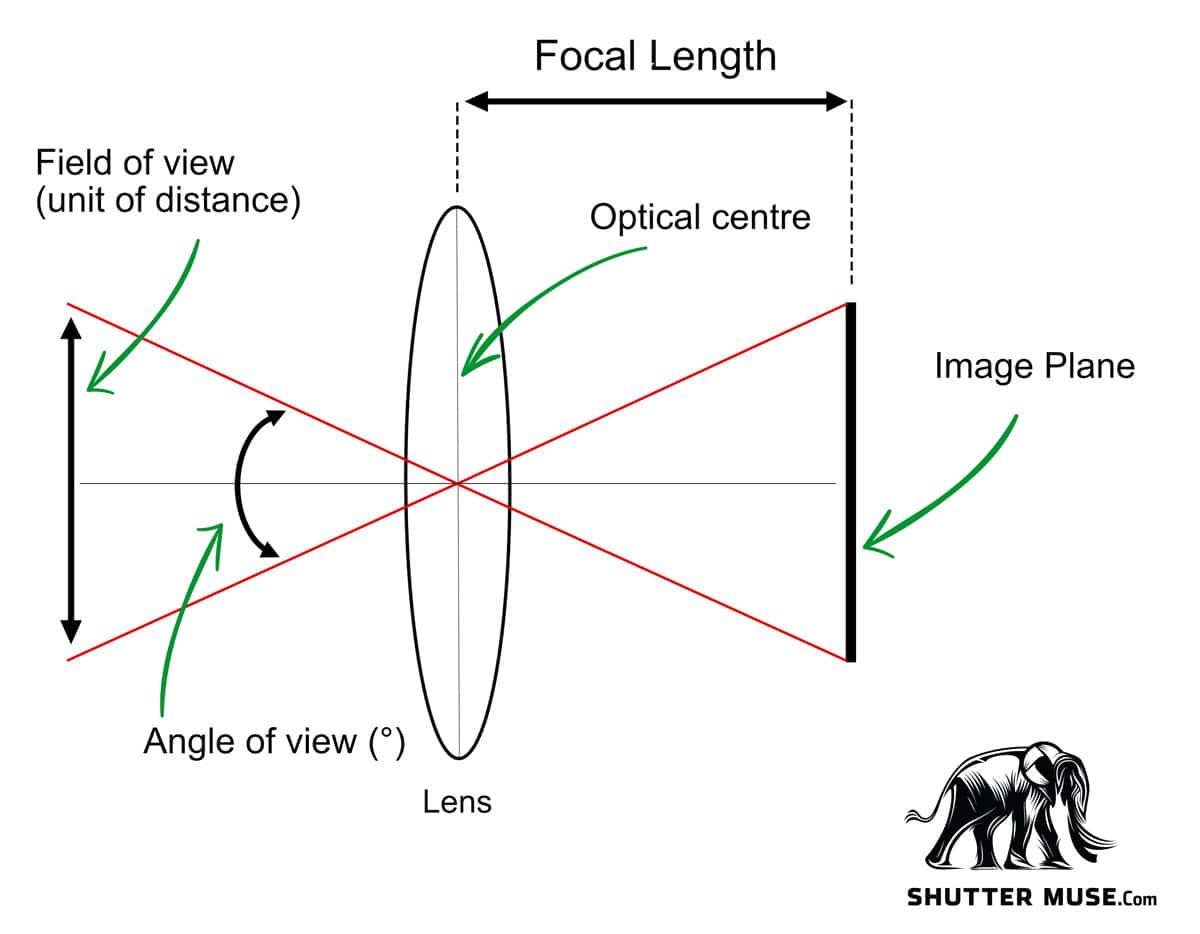
Angle of view (AOV)
Defined by the equation:
Angle of view (in degrees) = 2 ArcTan( sensor width / (2 X focal length)) * (180/π)
This is the formula that is most commonly cited for angle of view, and it agrees with the way in which lens specifications are presented by all the major camera manufacturers.
The angle of view is affected by both the focal length of the lens, and the size of the sensor in the camera. A long focal length delivers a very small angle of view. A short focal length delivers a very wide angle of view, hence the term “wide angle lens”. Here you can find tables of common angles of view for a variety of focal lengths and a little more about the math.
Field of view (FOV)
Defined by the equation:
Field of view = 2 (Tan (Angle of view/2) X Distance to Subject)
Field of view is a measurement of distance and it requires the knowledge of the distance from the lens’ optical centre to the subject. If you know the focal length, and the distance to subject, you can calculate the angle of view and then the field of view. This would allow you to calculate the size of something within your frame, or, in reverse you could calculate your distance to the subject if you knew the size of it and what proportion of your frame it filled.
One thing I discovered that backs up my theory that this is the best definition for field of view is that this is exactly how all binocular manufacturers use this terminology in binocular specifications. That industry uses the standardized distance of 1000yds as the distance to subject, and binoculars will often list a specification such as “Field of view: 300ft at 1000yds”. Source: allaboutbirds.org

Angular field of view (AFOV)
Exactly the same thing as angle of view.
Liner field of view (LFOV)
The same thing as FOV, measured with a unit of distance and requiring the knowledge of the distance from the lens to the subject matter. Most often used when also using the term AFOV. eg “The Canon 35mm lens has an angular field of view of 54.4 degrees and a linear field of view of 103ft at 1000yds”.
What do you think about this set of definitions?
Leave a comment below, but if you want to disagree with me then it would be wonderful if you could cite some sources because there are already far too many forum threads out there on this topic which have simply degraded into back-and-forth conversations taking the form of “I’m right” , “No, I’m right”. Let’s not have this become another one of those! I want to get to the bottom of this!







Those who have said “I’ll never use this stuff again” during Trig class, may be at a disadvantage grasping this article. The lesson is to stay open to all types of information because you really never know when it would be useful to your understanding of things.
Do you think I should explain the math a little bit more, Ed? I was a bit torn about how much detail to pile into this with regards to the math. Maybe I should bust out my SOH CAH TOA diagrams…
No I am afraid explaining the math would only repulse most folks (sorry). I think this article is an excellent example for a “math for artists” curriculum, say at RIT.
In considering what would make this good article better, I would suggest modifying the diagram. Currently, while mathematically correct, it is too abstract. It does not make the visceral connection that photographers have in the field with the difference between focal length and “field of view at 1000 yards”. I would suggest stretching the left side of the diagram to be longer. It does no have to be proportional, just suggest 200mm vs 1000 yds., or even 200mm vs 10yds. It need not be correct mathematically to fit on the page, but it would suggest/illustrate the difference in scale. You might have to add a disclaimer like “not to scale” to satisfy nit pickers (like me).
In responding to this, I flashed-back to my first photography class. The professor asked, “what is light?”. When it came to my turn, I said something like “electo-magnetic energy that is absorbed or reflected”. I was trying to appeal to the 1970’s “energy thing”, and still suggest how one understood exposing film and paper. But this completely missed the emotional aspect of photography as in a classmate’s response, “the absence of darknesss”. Her answer was far more abstract and liberating. For me the coolest thing about photography is that it embraces both worlds, the world of the scientist and the worl of the artist.
Thanks for the suggestion, I definitely see your point. I’ve always been fascinated by both the mathematical and artistic side of photography since my background, prior to being a professional photographer, was in aerospace engineering.
I was thinking the same thing about how binocular manufacturers refer to FOV as a function of distance. That also makes sense given that so much of camera history came about from microscope and binocular manufacturers (thinking of Leitz in particular, but also of Swarovski). I have to imagine that, had some of those mathematics junkies been born a century later, some of them would likely be aerospace engineers as well!
Great article.
Thank you! I’m glad you found it interesting!
Your final statement for definition of FOV makes perfect sense to me. If one was familiar with specifications for binoculars or rifle scopes one would find this familiar. FOV is a primary consideration when contemplating a purchase of either, in my view. Thus it’s a consideration in choosing focal lengths of camera lenses. Interesting post. Thanks. & BTW, I remember Whistler, where your bio says you live before there was any resort, just a dirt parking lot for a roadside cafe.
Glad you found it interesting! Whistler has changed a lot since then haha. That must have been quite some time ago. 70’s?
I found this article very useful on this topic and I think you will too!
http://panalab.panavision.com/sites/default/files/docs/documentLibrary/2%20Sensor%20Size%20FOV%20%283%29.pdf
Thanks for sharing that!
omg thank you – i stumbled on this this morning while i was desperately trying to understand the semantics myself!
Thanks “Anon”. We’re friendly here… you can use a name!
Thank you! You have explained these concepts much better than my university profs :/ Although, the equation that I was given for angle of view actually incorporates the pixel size {AOV = 2*arctan((sensor width/2*pixel size)/focal length)} … any ideas where the differences may stem from?
Interesting… how is “pixel size” defined? They are typically thought of as square, so is “size” the area? The width?
Thank you for your explanation obout FOV and AOV.But I have another confusion about FOV. In display industry,especially AR/VR,a key parameter is FOV that is different from what you said. It is showed as an angle,for example,which will be printed on the specification of VR/AR product,such as 210° showed by Sony.Meanwhile,there is another key parameter which is called AOV ( angle of view)of LCD or OLED screen. I just want to know the mathematical relationships between AOV and FOV what I said above. Thanks again!
I’m sorry, I have no experience with the AR/VR industry so I’m not the best person to ask about this one.
Measuring the angle of view the sketchy but instructive way: Take two knitting pens. Place them in de diagonal corners of the size of the sensor/film size – a 35 transparency frame is best. Take a key ring and move this up and down the two knitting pens, each pointing at the opposite corner. The point where they cross is the focal point. Move the crossing point to the focal distance of which you want to measure the angle of view. Take a geometric triangle and read the corresponding degrees. Within the transparency frame one might draw the various (sensor) formats and see what happens when placing one of the knitting pens to the other sizes of the “recording” surface.
Hi, your ‘angle of view’ calculation is surely for horizontal angle of view, whereas (as in your early quotes in the article) it usually refers to the diagonal angle of view. This matters because the aspect ration of an image varies (e.g., portrait vs. landscape).
Horizontal angle of view is normal, I’m not sure why Sony wants to measure things diagonally. As far as I’m concerned, that is way less useful!
Diagonal is usually used because it is meaningful whatever the aspect ratio of the sensor/image — e.g., 4:3, 3:2, 16:9 which are nowadays often settable in-camera. It also means you get the same value for a portrait image as for a landscape view. That’s useful. A lens doesn’t change it’s angle of view when you rotate the camera …
The sensor width doesn’t change, pi doesn’t change, focal length doesn’t change… I’m not sure why you think AOV is changing if you rotate the camera. You are just capturing the same angle of view in a different direction. The equation I have stated, goes hand in hand with the diagram. This partly depends on how people define sensor width, and whether they perceive the sensor to have the same width in vertical and horizontal orientation.
I believe most people by default would always take the width as the dimension that is parallel to the bottom of the camera. If you stood a car vertically up on it’s end and asked someone how long the car is, they would tell you the distance between the front and rear bumper. Even though that might technically be considered its height at that point.
I also think that people who are going to bother to read this article will have enough common sense to make the switch in width and height if they are calculating this in order to know how much horizontal view they will capture with the camera in the vertical orientation. They just have to follow the diagram.
Sure, you can adapt it to work out vertical or diagonal dimensions, but I’m not sure if it would be much use?
The world wraps around me for 360 degrees when I’m in the field. It’s much more practical for me to know how much of that 360 degrees can be viewed horizontally through a specific lens.
We’re going to have to agree to differ on this one; if someone asks me the width of a portrait photo I’m not going to respond with the height :-).
However it’s good to hear different views, and they have been food for thought. In fact I’ve changed my PanGazer program (http://speleotrove.com/pangazer/) to show both horizontal and vertical AOVs as part of the ‘size’ information for an image, while continuing to show diagonal AOV for the lens.
It’s Diagonal AOV which is IMO, not needed.
Nor is most of the math except for the really bored.
The CONCEPT is very easy to grasp.
AOV is measured in degrees from the point of the camera. Horizontal or vertiacl both give useful information for the aware photographer.
FOV is measured at the location of the subject and is measured in feet, meters, inches or furlongs. You could measure horizontla / vertical knowing if the camera is in portrait or landscape ‘hold’.
Binoculars have a regular system worked out for FOV so differing binoculars can easily be compared.
I was doing some programming today to get the angular field of view – i attempted the equation without knowing the diagonal. Turns out, in order to match spec from Nikon of a 20mm FF sensor, you NEED to know the diagonal because they measure it with the diagonal.
Here is the code:
https://www.online-python.com/8UpXS4xgkq
Imagine yourself standing in front of a window. Your head is aligned so that the center point of the window is aligned with the center point between your eyes.
You take a step closer to the window. The field of view is the same but your angle of view is greater thus you can see more because you “widened” your angle of view. As you can’t get anything for nothing the trade off is the more you can see the more distorted it is.circle become ovals strait lines appear curved ect.
Now as you stand there someone cut out the window leaving a hole . Your field of view widened so you can see more but your angle of view basically stayed the same. The trade off for this is the effort it took or money spent cutting out the window. Or for humans it’s the effort it took your mom to form your eyeball in her womb.
Excellent!
This cleared out lot of doubts, im implementing an automatic detection of AOV with canon edsdk and this was pretty useful.
Thanks Dan!
you’re very welcome
I truly and greatly appreciate the information provided here both in the article and the comments.
This is my opinion (which is somewhat different from yours):
Summary:
*AOV and FOV are definitely not the same.
*When a lot of people (including camera manufacturers) speak about AOV they are actually talking about FOV.
*AOV is an inherent characteristic of a particular lens which is not alterable.
*FOV is a property resulted from a combination of the characteristics of a lens and the film/sensor format.
Elaboration:
I agree with the various sources cited in the article and the comment section that AOV and FOV, though related, are not the same and, hence, cannot and should not be used interchangeably.
My understanding is that AOV describes how much of the physical the lens covers. In the simplest case of a single-element lens, AOV is dependent on the focal length alone. In a multiple-element lens, it is dependent on not just the focal length but also the particular design of the lens — that is why different lenses of the same focal length can have a slightly different AOV.
While AOV describes how much of the physical scene the lens covers, FOV refers to how much of the lens’ Image Circle (IC) the film/sensor covers. AOV should not be used to explain Crop Factor (CF), even though many sources do, including camera manufacturers. Instead, FOV should be used because, quite literally, the existence of CF is a result from the fact that different formats (size and shape) of sensor cover different amount of the IC — this is, of course, the definition of FOV.
I have the suspicion that camera manufacturers use AOV (instead of the correct concept of FOV) to explain CF is because it is simply easier than to introduce to the consumers yet another term and to spend time explaining the difference. For the same reason, I suspect, they use the misconception of “full frame equivalent focal length” when they know full well that the focal length of a lens won’t change because you put it on cameras with different sensor formats. And the reason camera manufacturers do it is not necessarily as nefarious as it may appear. I believe that camera manufacturers do it simply because most consumers are not interested and do not possess the basic knowledge to understand the nuances of the science and technology behind photography. Thus, a simpler, albeit inaccurate, explanation is more palatable to and digestible for most consumers.
Thanks for adding this detailed analysis! Definitely some good points made here Tom.
Thank you for you well researched presentation.
Yes, there is a lot of confusion regarding both Angle of View (AOV) and Field of View (FOV). I appreciate your considerable effort to bring some clarity to the subject. AOV is fairly straight forward but misused and FOV is ambiguous – and misused.
First of all, AOV is a specification of a lens. The AOV of a lens is based on the lens focused to infinity using the sensor (or film) size for which it was designed. It is the angular extent of the scene that is captured – for that sensor, at infinity. If looking at a lens irregardless of sensor or film, the correct term for what the lens can transmit is angle of coverage – a term that large format photographers have to use when describing a lens’ capabilities unrelated to the back plane.
Field of view simply means that which can be seen from a specific vantage point. The ambiguity of FOV comes from the fact that such a view can be described both in terms of angles and dimensions. FOV refers to the scene to be captured which infers focus – a specific distance from the camera, consequently FOV has as a component the distance to the plane of focus. It is the lens in use. For most non-cinema lenses, as focus changes so does the FOV. Photographers refer to this as “breathing” – most lenses for photography allow some breathing while cinema lenses generally do not. As an example, when we do focus stacking by changing focus, we ever so slightly also change the FOV for each frame, even with a fixed focal length prime lens [this is why focusing by moving the camera is better].
AOV describes the lens and just like saying that an f/2.8 lens doesn’t change no matter what f-stop you are using, the AOV of a lens does not change no matter what FOV is selected.
Describing the FOV as angles is the Angular Field of View (AFOV), describing FOV as dimensions (width and height) is the Linear Field of View (LFOV) but adding such esoteric terms to a presentation that is meant to clarify may not be helpful. Bottom-line is when the lens has been focused to infinity the angular FOV equals the AOV just like what occurs with f-stops when we fully open up our aperture.
The AOV does not equal the angle of coverage or image circle. For example, Canon states that all of their 50mm lenses have an AOV (diagonal) of 46°, even their TSE-50mm! For the TSE-50mm to accomplish shifts, its angle of coverage has to be significantly larger than 46° probably closer to 64°. Because the AOV that manufacturers identify for a lens directly relates to the size of the sensor, using the crop factor works well when determining AOV when working with other sensor sizes.
Consider having a diagram just for AOV. If you want to add clarity to the term, limit its use to the lens specification and remove the reference to “distance.” You might also show the “Angle of view” caption on the opposite side of the lens (the space in front of the sensor) as it refers to what is captured at infinity rather than what the lens is transmitting. And, you could show that the lens is focused to infinity. Indicate that this is a lens specification.
A second diagram for FOV would be a duplicate of the AOV diagram with the “Field of view” (or Linear field of view if you must) caption referencing dimensions (rather than distance) included and caption “Angular field of view” in front of the image plane. You could show that change in focus changes AFOV and dimensions.
The FOV (angular and linear) refer to the lens set to a specific focus, not necessarily infinity. FOV describes what is captured at a given focus.
By the way AFOV is also regularly used to refer to apparent field of view and actual field of view.
Does this reduce confusion? A little, for some. But the technical aspects, let alone the terminology, has little to do with the joy of photography and the sense of creativity experienced with a great capture.
Michael, I just wanted to acknowledge your comment. Thank you so much for taking the time to explain all this. I’m on Christmas vacation, but when I get back I’ll take some more time to digest this. I just wanted to reply with a quick thanks right now! Cheers!
Great Article, Thanks to clear the doubts. This will help me in my projects
You’re welcome!
NOW i understand and thank you. This plagued my for forever. Now I can move passed this issue
Great to hear!
Good Article. I’m a practicing wildlife and fashion photographer. I bought many lenses from Year 2016. But i can say, from year 2018 beginning, i started noticing AOV of the lenses in its specification, especially when i looked into buying higher focal length. I have been using Canon 80D which is of consisting 1.6x CF sensor.
From my observation, On a FF Camera sensor, a single digit (say 7 degree or 8 degree) AOV lenses with higher focal length (400 & above) lenses produces exclusively very good sharp subject in IC, leaving rest all elements in OOF. It means FOV is with very shallow DoF with its widest aperture.
When i use same lens in my CF camera body, FOV changes as the scene covers other elements too with the subject in focus, set at its widest aperture.
In my opinion, the FOV changes with respect to its sensor size of the camera. In other words, Sensor size of the camera does really directly proportional to the changes in FOV (i.e subject in focus).
Most of the wildlife photographers, do shoot at natural light environment. Therefore, they intend to gather more light to sensor. This action results them to keep lenses operate at its widest aperture of the lens manufacturer and rarely they narrow down if there is very much bright light available at that area/land. So, the FOV does matter a lot as the subject isolation from the background is to be achieved in this kind of photography.
Dear All,
Thanks for sharing knowledge regarding this. I have one question. I have 2 images and have stitched them. Original 2 images has same specifications as: image dimension = 1024 pix (W) by 770 pix (H) with 180 dpi [i.e. 144 mm (W) by 108 mm (H)], Camera focal length 35 mm, lens focal length 9 mm, max. aperture 4, F-stop f/4, exposure time 1/100 sec. Now stitched image dimension is 1600 pix (W) by 900 pix (H) with 96 dpi [i.e. 423 mm (W) by 238 mm (H)]. Only these parameters known. In above case what is FOV (in distance and in angle) of original and stitched image? Please acknowledge.
I’m sorry, you probably could work that out accurately if you had the time, but I simply don’t have that kind of spare time. It’s hard enough to simply give quick answers to all the comments on this site. Why don’t you just find out the angle of view for one image and then multiply by two and factor in some amount of overlap. That would give you a rough answer quickly.
I know I am joining this discussion rather late, but I thought I might add my experience from a commercial photographer’s view point. AOV & FOV are different, both have affect composition in vastly different ways and are confused by many, including lens makers.
The easiest way I think about the difference (in the most simplest sense) is to photograph a subject matter with a zoom lens with lots of foreground and background showing. If you wanted to fill the subject matter completely in the frame and if you zoom in, that is altering the AOV. If you walk up to the subject matter, keeping the same focal length on the zoom and fill the frame you are changing the FOV. The latter also applies to cropped sensors (sorry Nikon, Et al) and cropping an image.
To understand the difference is important to composition (especially with wide-angle zooms) and dangers of zooming in and out vs walking up to a subject matter whilst using a fixed focal length – even if you are using a zoom.
I am strong believer in learning on different fixed focal lengths lenses as it teaches you the discipline of moving around a subject matter to achieve composition. Even on a photo shoot while using a zoom for speed and convenience, I have to fight the urge to zoom in or out rather than think about a different way to achieve a certain look. Especially in situations where there are back ground distractions or space is tight and you foolish opt for the widest focal length on your zoom.
Thanks so much for the insightful comment. This is definitely food for thought. In fact I think I’ll move this comment up to the foot of the content so everyone can read it. I love the discussion that this topic has generated!! Cheers for contributing.
I have been studying this for a while. I think the important issue is the affect of angle of view in the images. It is very important and often neglected. The angle of view is the reason your portraits of faces at 35mm lens are distorted from the same shot at the same size with an 85mm lens. If you look at a full body portrait on a 35mm lens you will see the too of the shoe is very long. On the 85mm you see more of the front of the shoe. 135mm is my favorite portrait lens because the angle of view give a more realist and flattering reproduction of what I saw when I took the picture.
Hi Dan,
Thank you for the post. I have come across it when I was confused about different interpretations/uses of the Field of View/Angle of View/Instantaneous Field of View etc.
Now, let me lay out my understanding based on some research I have conducted and share it with everyone else.
Imagine Im flying at a height of 3km above the ground with a camera that has 9.6mm detector width (sensor’s width) and a field lens (objective/seeing/telescope whatever it might be called anywhere) that has 30mm focal length.
Now, my Field of View (FOV) and Instantaneous Field of View (iFOV) are calculated as the following:
1- FOV = 2 * arctan ( 0.5 * sensor width / focal length ) = 18.18 degrees
2- iFOV = FOV / # of pixels = 18.18 degrees / 640 pixels = 0.0284 degrees = 0.495 Milliradian
Note: The iFOV is the angle subtended by the detector element (one pixel). This calculation is for the Horizontal axis.
I hope Im not adding to the confusion already.
P.S: This is from a scientific/physics remote sensing prospective.
Cheers,
Naif Alsalem
The angle of view describes the geometry of the lens. Field of view describes the scene on the camera sensor. On a wide Angle lens the angle of view remains the same but your field of view will change as you move closer or farther from the subject. Angle of view also can add unwanted distortion on very wide angle lenses which is why most portrait lenses are traditionally 85-135mm.
That’s a nice way to define the differences. Thanks for sharing.
Good information over again. Thanks=)